1. Applegate, D., Bixby, R., Chvatal, V., Cook, W.: On the Solution of Traveling Salesman Problems. Documenta Mathematica (Journal der Deutschen Mathematiker-Verinigung, International Congress of Mathematicians), 645–656 (1998)Google Scholar
2. Ball, B.C., Webster, D.B.: Optimal Scheduling for Even-Numbered Team Athletic Conferences. AIIE Trans. 9, 161–169 (1977)Google Scholar
3. Barnhart, C., Johnson, E.L., Nemhauser, G.L., Savelsbergh, M.W.P., Vance, P.H.: Branch-And-Price: Column Generation for Huge Integer Programs. Oper. Res. 46, 316–329 (1998)zbMATHCrossRefMathSciNetGoogle Scholar
4. Benoist, T., Laburthe, F., Rottembourg, B.: Lagrange Relaxation and Constraint Programming Collaborative Schemes for Traveling Tournament Problems. In: CPAIOR, Wye College, pp. 15–26 (2001)Google Scholar
5. Easton, K.: Using Integer and Constraint Programming to Solve Sports Scheduling Problems. Doctoral Dissertation, Georgia Institute of Technology, Atlanta (2002) Google Scholar
6. Easton, K., Nemhauser, G.L., Trick, M.A.: The Traveling Tournament Problem: Description and Benchmarks, Principle and Practices of Constraint Programming. In: Walsh, T. (ed.) CP 2001. LNCS, vol. 2239, pp. 580–585. Springer, Heidelberg (2001)CrossRefGoogle Scholar
7. Gabow, H.N.: Data Structures for Weighted Matching and Nearest Common Ancestors with Linking. In: Proc. 1st Annu. ACM–SIAM Symp. Discr. Algorithms, pp. 434–443. SIAM, Philadelphia (1990)Google Scholar
8. Henz, M.: Scheduling a Major College Basketball Conference: Revisted. Oper. Res. 49, 163–168 (2001)zbMATHCrossRefMathSciNetGoogle Scholar
9. Kirkpatrick, D.G., Hell, P.: On the Complexity of a Generalized Matching Problem. In: Proc. 10th Annu. ACM Symp. Theory Computing. Association for Computing Machinery, New York, pp. 240–245 (1978)Google Scholar
10. Klabjan, D., Johnson, E.L., Nemhauser, G.L.: Solving Large Airline Crew Scheduling Problems: Random Pairing Generation and Strong Branching. Comput. Optim. Appl. 20, 73–91 (2001)zbMATHCrossRefMathSciNetGoogle Scholar
11. Russell, R.A., Leung, J.M.: Devising a Cost Effective Schedule for a Baseball League. Oper. Res. 42, 614–625 (1994)zbMATHCrossRefGoogle Scholar

Diverse group is the best solution for problem-solving tasks By Jared Wadley News Service. A diverse group of problem solvers is more likely to outperform a team of the best and brightest problem solvers, a U-M study shows. Individuals chosen from a diverse, randomly selected pool will offer different perspectives that could result in better. Chapter 1 – Problem Solving with Math 141. Multiply (round solution to nearest hundredth): 9.158 14.382 142. Divide (round to nearest tenth): 118,000 3.95 143. Complete by shortcut method: 43.81 1,000 144. Complete by shortcut method: 11,896.413 1,000 145. Complete by shortcut method: 3,069.44 1,000 146. Mel Doane is taking his office staff. Go Math Grade 4 Answer Key Chapter 1 Place Value, Addition, and Subtraction to One Million contains 4th Standard Go Math solutions which help the students to score well in the exams.This Go Math Grade 4 Answer Key Chapter 1 Place Value, Addition, and Subtraction to One Million will make students understand the concepts easily. In this, each and every question was explained intimately.

Diverse group is the best solution for problem-solving tasks

A diverse group of problem solvers is more likely to outperform a team of the best and brightest problem solvers, a U-M study shows.

Individuals chosen from a diverse, randomly selected pool will offer different perspectives that could result in better solutions. Conversely, a group comprising the best problem solvers is likely to take similar approaches, says Scott Page, a political science, economics and complex systems professor.

'If the best problem solvers tend to think about a problem similarly, then it stands to reason that as a group, they may not be very effective,' he says.

Page conducted the research with Lu Hong, a visiting professor in the Stephen M. Ross School of Business and a faculty member at Loyola University in Chicago. The Proceedings of the National Academy of Sciences published the paper this month.

In the study, diversity wasn't necessarily meant to indicate identity diversity—differences in race, gender, age or life experiences—but differences in how problem solvers encode problems and attempt to solve them. A person's value to solving problems depends on his or her ability to improve the collective decision, the researchers say.

'A person's expected contribution is contextual, depending on the perspectives and heuristics of others who work on the problem,' says Page, who also is a senior research scientist at the Institute for Social Research. Heuristics are the variations in how people encode and search for solutions to problems.

Page and Hong tested their theory using computational and mathematical models, with each determining the best performance occurred when the problem-solving group was diverse.

Page notes that the collection of problem solvers could sit in a room together making a joint decision. These individuals might operate in a hierarchy, in which each person works on a problem and passes his or her solution on to the next person, he says.

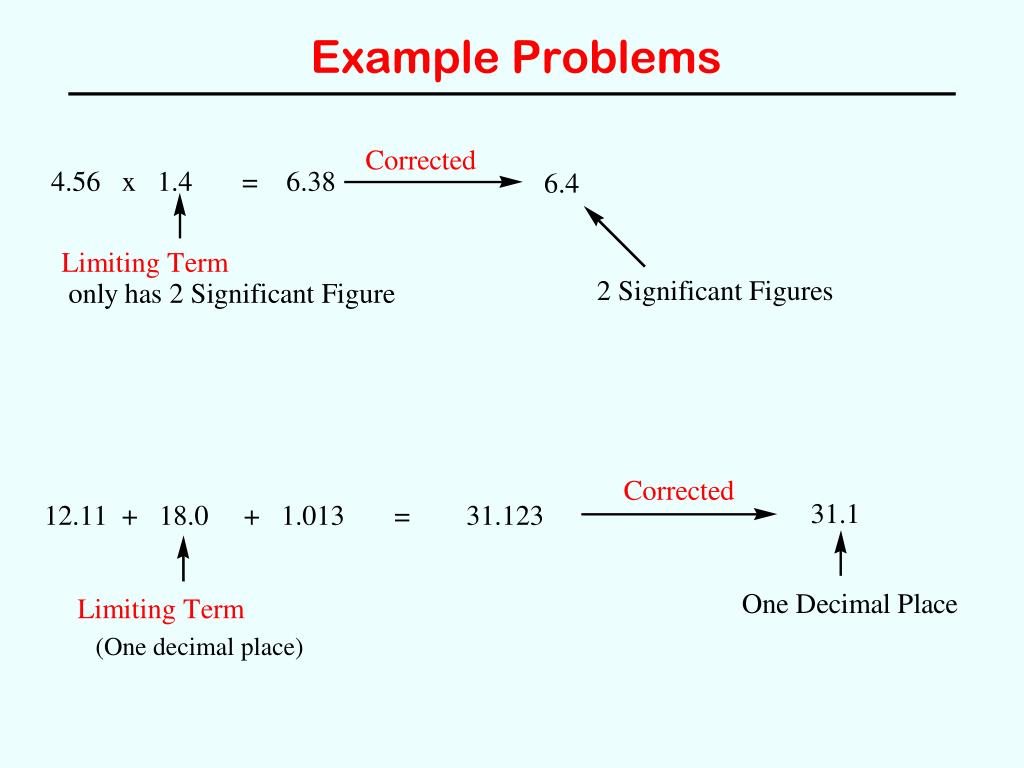
Solving Math Equations

The researchers say the study's results have implications for organizational forms and management styles, especially for problem-solving firms and organizations.

'In an environment where competition depends on continuous innovation and introduction of new products, firms that take advantage of the power of functional diversity should perform well,' Hong says.

Page teaches an undergraduate course, 'Theories of Diversity,' that focuses on the many implications of diversity. His course touches on topics ranging from the stability of political systems and ecosystems to the collective wisdom of crowds.

Math Problem Solving

For more information on Page and the project, visit http://www.cscs.umich.edu/diversity/.